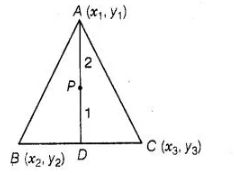
The points A(x1, y1), B(x2 y2) and C(x3, y3)

Given that, the points A (x1, y1), B(x2, y2)andC (x3, y3)are the vertices of ΔABC.

(i) We know that, the median bisect the line segment into two equal parts i.e., here D is the mid-point of BC.

$\therefore$ Coordinate of mid-point of $B C=\left(\frac{x_{2}+x_{3}}{2}, \frac{y_{2}+y_{3}}{2}\right)$

$\Rightarrow$ $D \equiv\left(\frac{x_{2}+x_{3}}{2}, \frac{y_{2}+y_{3}}{2}\right)$

(ii) Let the coordinates of a point $P$ be $(x, y)$. 

Given that, the point $P(x, y)$, divide the line joining $A\left(x_{1}, y_{1}\right)$ and $D\left(\frac{x_{2}+x_{3}}{2}, \frac{y_{2}+y_{3}}{2}\right)$ in

the ratio $2: 1$, then the coordinates of $P$

$=\left[\frac{2 \cdot\left(\frac{x_{2}+x_{3}}{2}\right)+1 \cdot x_{1}}{2+1}, \frac{2 \cdot\left(\frac{y_{2}+y_{3}}{2}\right)+1 \cdot y_{1}}{2+1}\right]$

$\left[\because\right.$ internal section formula $\left.=\left(\frac{m_{1} x_{2}+m_{2} x_{1}}{m_{1}+m_{2}}, \frac{m_{1} y_{2}+m_{2} y_{1}}{m_{1}+m_{2}}\right)\right]$

$\equiv\left(\frac{x_{2}+x_{3}+x_{1}}{3}, \frac{y_{2}+y_{3}+y_{1}}{2}\right)$

$\therefore$ So, required coordinates of point $P \equiv\left(\frac{x_{1}+x_{2}+x_{3}}{3}, \frac{y_{1}+y_{2}+y_{3}}{3}\right)$

(iii) Let the coordinates of a point $Q$ be $(p, q)$

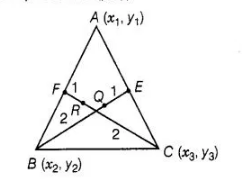
Given that, the point $Q(p, q)$, divide the line joining $B\left(x_{2}, y_{2}\right)$ and $E\left(\frac{x_{1}+x_{3}}{2}, \frac{y_{1}+y_{3}}{2}\right)$ in

the ratio $2: 1$, then the coordinates of $Q$

$\equiv\left[\frac{2 \cdot\left(\frac{x_{1}+x_{3}}{2}\right)+1 \cdot x_{2}}{2+1}, \frac{2 \cdot\left(\frac{y_{1}+y_{2}}{2}\right)+1 \cdot y_{2}}{2+1}\right]$

$=\left(\frac{x_{1}+x_{2}+x_{3}}{3}, \frac{y_{1}+y_{2}+y_{3}}{3}\right)$

$[$ since, $B E$ is the median of side $C A$, so $B E$ divides $A C$ in to two equal parts. 

$\therefore$ mid-point of $A C=$ Coordinate of $\left.E \Rightarrow E=\left(\frac{x_{1}+x_{3}}{2}, \frac{y_{1}+y_{3}}{2}\right)\right]$

So, the required coordinate of point $Q \equiv\left(\frac{x_{1}+x_{2}+x_{3}}{3}, \frac{y_{1}+y_{2}+y_{3}}{3}\right)$

Now, let the coordinates of a point $E$ be $(\alpha, \beta)$. Given that, the point $R(\alpha, \beta)$, divide the line

joining $C\left(x_{3}, y_{3}\right)$ and $F\left(\frac{x_{1}+x_{2}}{2}, \frac{y_{1}+y_{2}}{2}\right)$ in the ratio $2: 1$, then the coordinates of $R$

$\equiv\left[\frac{2 \cdot\left(\frac{x_{1}+x_{2}}{2}\right)+1 \cdot x_{3}}{2+1}, \frac{2 \cdot\left(\frac{y_{1}+y_{2}}{2}\right)+1 \cdot y_{3}}{2+1}\right]$

$=\left(\frac{x_{1}+x_{2}+x_{3}}{3}, \frac{y_{1}+y_{2}+y_{3}}{3}\right)$

[ since, $C F$ is the median of side $A B$. So, $C F$ divides $A B$ in to two equal parts. 

$\therefore$ mid-point of $A B=$ coordinate of $\left.F \Rightarrow F=\left(\frac{x_{1}+x_{2}}{2}, \frac{y_{1}+y_{2}}{2}\right)\right]$

So, the required coordinate of point $R \equiv\left(\frac{x_{1}+x_{2}+x_{3}}{3}, \frac{y_{1}+y_{2}+y_{3}}{3}\right)$

(iv) Coordinate of the centroid of the $\triangle A B C$

$=\left(\frac{\text { Sum of abscissa of all vertices }}{3}, \frac{\text { Sum of ordinate of all vertices }}{3}\right)$

$=\left(\frac{x_{1}+x_{2}+x_{3}}{3}, \frac{y_{1}+y_{2}+y_{3}}{3}\right)$