The set of all possible values of θ in the interval

Given that both points $(1,2) \&(\sin \theta, \cos \theta)$ li on same side of the line $x+y-1=0$

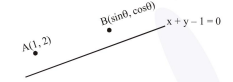
So, $\left(\begin{array}{l}\text { Put }(1,2) \text { in } \\ \text { given line }\end{array}\right)\left(\begin{array}{l}\text { Put }(\sin \theta, \cos \theta \text { in } \\ \text { given line }\end{array}\right)>0$

$\Rightarrow(1+2-1)(\sin \theta+\cos \theta-1)>0$

$\Rightarrow \sin \theta+\cos \theta>1\{\div$ by $\sqrt{2}\}$

$\Rightarrow \frac{1}{\sqrt{2}} \sin \theta+\frac{1}{\sqrt{2}} \cos \theta>\frac{1}{\sqrt{2}}$

$\Rightarrow \sin \left(\theta+\frac{\pi}{4}\right)>\frac{1}{\sqrt{2}}$

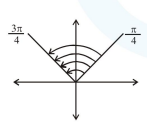
$\Rightarrow \frac{\pi}{4}<\theta+\frac{\pi}{4}<\frac{3 \pi}{4}$

$\Rightarrow 0<\theta<\frac{\pi}{2}$