The sides of a quadrilateral ABCD are 6 cm,

Given ABCD is a quadrilateral having sides AB = 6 cm, BC = 8 cm, CD = 12 cm and DA = 14 cm. Now, join AC.

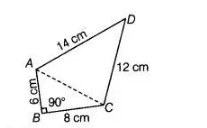
We have, $A B C$ is a right angled triangle at $B$.

Now, $\quad A C^{2}=A B^{2}+B C^{2} \quad$ [by Pythagoras theorem]

$=6^{2}+8^{2}=36+64=100$

$\Rightarrow \quad A C=10 \mathrm{~cm} \quad$ [taking positive square root]

$\therefore$ Area of quadrilateral $A B C D=$ Area of $\triangle A B C+$ Area of $\triangle A C D$ $\ldots$ (i)

Now, area of $\triangle A B C=\frac{1}{2} \times A B \times B C$ $\left[\because\right.$ area of triangle $=\frac{1}{2}($ base $\times$ height $\left.)\right]$

$=\frac{1}{2} \times 6 \times 8=24 \mathrm{~cm}^{2}$

In $\triangle A C D$ $A C=a=10 \mathrm{~cm}, C D=b=12 \mathrm{~cm}$

and $D A=C=14 \mathrm{~cm}$

Now, semi-perimeter of $\triangle A C D, s=\frac{a+b+c}{2}=\frac{10+12+14}{2}=\frac{36}{2}=18 \mathrm{~cm}$

Area of $\Delta A C D=\sqrt{s(s-a)(s-b)(s-c)}$ [by Heron's formula]

$=\sqrt{18(18-10)(18-12)(18-14)}$

$=\sqrt{18 \times 8 \times 6 \times 4}=\sqrt{(3)^{2} \times 2 \times 4 \times 2 \times 3 \times 2 \times 4}$

$=3 \times 4 \times 2 \sqrt{3 \times 2}=24 \sqrt{6} \mathrm{~cm}^{2}$