The triangle of maximum

$h=r \sin \theta+r$

base $=\mathrm{BC}=2 \mathrm{r} \cos \theta$

$\theta \in\left[0, \frac{\pi}{2}\right)$

Area of $\triangle \mathrm{ABC}=\frac{1}{2}(\mathrm{BC}) \cdot \mathrm{h}$

$\Delta=\frac{1}{2}(2 r \cos \theta) \cdot(r \sin \theta+r)$

$=r^{2}(\cos \theta) \cdot(1+\sin \theta)$

$\frac{\mathrm{d} \Delta}{\mathrm{d} \theta}=\mathrm{r}^{2}\left[\cos ^{2} \theta-\sin \theta-\sin ^{2} \theta\right]$

$=r^{2}\left[1-\sin \theta-2 \sin ^{2} \theta\right]$

$=\underbrace{\mathrm{r}^{2}[1+\sin \theta]}_{\text {positive }}[1-2 \sin \theta]=0$

$\Rightarrow \theta=\frac{\pi}{6}$

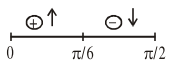
$\Rightarrow \Delta$ is maximum where $\theta=\frac{\pi}{6}$

$\Delta_{\max .}=\frac{3 \sqrt{3}}{4} \mathrm{r}^{2}=$ area of equilateral $\Delta$ with

$\mathrm{BC}=\sqrt{3} \mathrm{r}$