Question.
Tick the correct answer and justify : In $\Delta \mathrm{ABC}, \mathrm{AB}=6 \sqrt{3} \mathrm{~cm}, \mathrm{AC}=12 \mathrm{~cm}$ and $\mathrm{BC}=6 \mathrm{~cm}$. The angle $\mathrm{B}$ is :
(1) 120°
(2) 60°
(3) 90°
(4) 45°
Tick the correct answer and justify : In $\Delta \mathrm{ABC}, \mathrm{AB}=6 \sqrt{3} \mathrm{~cm}, \mathrm{AC}=12 \mathrm{~cm}$ and $\mathrm{BC}=6 \mathrm{~cm}$. The angle $\mathrm{B}$ is :
(1) 120°
(2) 60°
(3) 90°
(4) 45°
Solution:
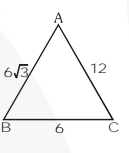
$\mathrm{AB}^{2}=(6 \sqrt{3})^{2}=108$
$\mathrm{BC}^{2}=6^{2}=36$
$A C^{2}=12^{2}=144$
So, $\mathrm{AB}^{2}+\mathrm{BC}^{2}=\mathrm{AC}^{2}$
$\Delta \mathrm{ABC}$ is right $\Delta$, right angled at $\mathrm{B}$
$\angle \mathrm{B}=90^{\circ}$

$\mathrm{AB}^{2}=(6 \sqrt{3})^{2}=108$
$\mathrm{BC}^{2}=6^{2}=36$
$A C^{2}=12^{2}=144$
So, $\mathrm{AB}^{2}+\mathrm{BC}^{2}=\mathrm{AC}^{2}$
$\Delta \mathrm{ABC}$ is right $\Delta$, right angled at $\mathrm{B}$
$\angle \mathrm{B}=90^{\circ}$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
