To draw a pair of tangents to a circle, which are inclined to each other at angle of 450 ,

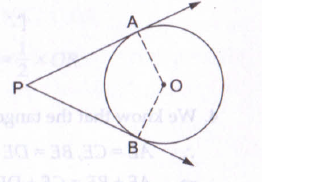
Suppose PA and PB are two tangents we want to draw which inclined at an angle of 450
We know that the radius and tangent are perperpendular at their point of contact
∵∠OBP = ∠OAP = 900
Now, In quadrilateral AOBP
∠AOB + ∠OBP +  + ∠OAP + ∠APB = 3600            [Angle sum property of a quadrilateral]
⇒ ∠AOB + 900 + 900 + 450 = 3600 
⇒ ∠AOB + 2250 = 3600 
⇒ ∠AOB = 1350 
Hence, the correct answer is option (b).