Two identical cubes each of volume 64 cm3

Let the length of side of a cube = a cm

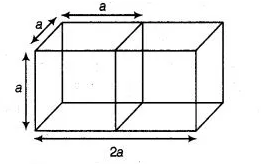
Given, volume of the cube, $a^{3}=64 \mathrm{~cm}^{3} \Rightarrow a=4 \mathrm{~cm}$ On joining two cubes, we get a cuboid whose

length, $l=2 \mathrm{acm}$

breadth, $b=a \mathrm{~cm}$

and height, $h=a \mathrm{~cm}$

Now, surface area of the resulting cuboid $=2(l b+b h+h l)$

$=2(2 a \cdot a+a \cdot a+a \cdot 2 a)$

$=2\left(2 a^{2}+a^{2}+2 a^{2}\right)=2\left(5 a^{2}\right)$

$=10 a^{2}=10(4)^{2}=160 \mathrm{~cm}^{2}$