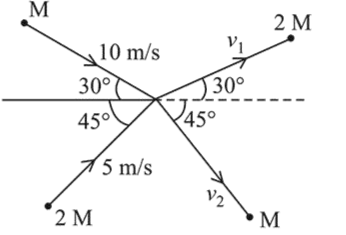
Two particles, of masses $\mathrm{M}$ and $2 \mathrm{M}$, moving, as shown, with speeds of $10 \mathrm{~m} / \mathrm{s}$ and $5 \mathrm{~m} / \mathrm{s}$, collide elastically at the origin. After the collision, they move along the indicated directions with speeds $\mathrm{v}_{1}$ and $\mathrm{v}_{2}$, respectively. The values of $v_{1}$ and $v_{2}$ are nearly :
Correct Option: 1
(1) Apply concervation of linear momentum in $X$ and $Y$ direction for the system then
$M\left(10 \cos 30^{\circ}\right)+2 M\left(5 \cos 45^{\circ}\right)=2 M\left(v_{1} \cos 30^{\circ}\right)$$+\mathrm{M}\left(\mathrm{v}_{2} \cos 45^{\circ}\right)$
$5 \sqrt{3}+5 \sqrt{2}=\sqrt{3} \mathrm{v}_{1}+\frac{\mathrm{v}_{2}}{\sqrt{2}}$ ..(1)
Also
$2 \mathrm{M}\left(5 \sin 45^{\circ}\right)-\mathrm{M}\left(10 \sin 30^{\circ}\right)=2 \mathrm{Mv}_{1} \sin 30^{\circ}-\mathrm{Mv}_{2} \sin 45^{\circ}$
$5 \sqrt{2}-5=\mathrm{v}_{1}-\frac{\mathrm{v}_{2}}{\sqrt{2}}$ ..(2)
Solving equation (1) and (2)
$(\sqrt{3}+1) \mathrm{v}_{1}=5 \sqrt{3}+10 \sqrt{2}-5 \Rightarrow \mathrm{v}_{1}=6.5 \mathrm{~m} / \mathrm{s}$
$\mathrm{v}_{2}=6.3 \mathrm{~m} / \mathrm{s}$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
