Two planets have masses $\mathrm{M}$ and $16 \mathrm{M}$ and their radii are a and $2 \mathrm{a}$, respectively. The separation between the centres of the planets is $10 \mathrm{a}$. A body of mass $m$ is fired from the surface of the larger planet towards the smaller planet along the line joining their centres. For the body to be able to reach at the surface of smaller planet, the minimum firing speed needed is :
Correct Option: , 2
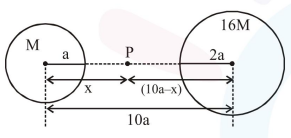
$\frac{\mathrm{GM}}{\mathrm{x}^{2}}=\frac{\mathrm{G}(16 \mathrm{M})}{(10 \mathrm{a}-\mathrm{x})^{2}}$
$\frac{1}{x}=\frac{4}{(10 a-x)} \quad \Rightarrow 4 x=10 a-x$
$x=2 a$ ...(1)
COME
$-\frac{\mathrm{GMm}}{8 \mathrm{a}}-\frac{\mathrm{G}(16 \mathrm{M}) \mathrm{m}}{2 \mathrm{a}}+\mathrm{KE}$
$=-\frac{\mathrm{GMm}}{2 \mathrm{a}}-\frac{\mathrm{G}(16 \mathrm{M}) \mathrm{m}}{8 \mathrm{a}}$
$\mathrm{KE}=\mathrm{GMm}\left[\frac{1}{8 \mathrm{a}}+\frac{16}{2 \mathrm{a}}-\frac{1}{2 \mathrm{a}}-\frac{16}{8 \mathrm{a}}\right]$
$\mathrm{KE}=\mathrm{GMm}\left[\frac{1+64-4-16}{8 \mathrm{a}}\right]$
$\frac{1}{2} \mathrm{mv}^{2}=\mathrm{GMm}\left[\frac{45}{8 \mathrm{a}}\right]$
$\mathrm{V}=\sqrt{\frac{90 \mathrm{GM}}{8 \mathrm{a}}}$
$\mathrm{V}=\frac{3}{2} \sqrt{\frac{5 \mathrm{GM}}{\mathrm{a}}}$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
