Question:
Two stars of masses $\mathrm{m}$ and $2 \mathrm{~m}$ at a distance $\mathrm{d}$ rotate about their common centre of mass in free space. The period of revolution is :
Correct Option: , 2
Solution:
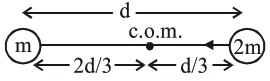
$\mathrm{F}=\frac{\mathrm{G}(2 \mathrm{~m}) \mathrm{m}}{\mathrm{d}^{2}}=(2 \mathrm{~m}) \omega^{2}(\mathrm{~d} / 3)$
$\frac{\mathrm{Gm}}{\mathrm{d}^{2}}=\omega^{2} \frac{\mathrm{d}}{3}$
$\Rightarrow \omega^{2}=\frac{3 \mathrm{Gm}}{\mathrm{d}^{3}}$
$\Rightarrow \omega=\sqrt{\frac{3 \mathrm{Gm}}{\mathrm{d}^{3}}}$
$\Rightarrow \mathrm{T}=\frac{2 \pi}{\omega}=2 \pi \sqrt{\frac{\mathrm{d}^{3}}{3 \mathrm{Gm}}}$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
