When a body slides down from rest along a smooth inclined plane making an angle of $30^{\circ}$ with the horizontal, it takes time $\mathrm{T}$. When the same body slides down from the rest along a rough inclined plane making the same angle and through the same distance, it takes time $\alpha \mathrm{T}$, where $\alpha$ is a constant greater than 1. The co-efficient of friction between
the body and the rough plane is $\frac{1}{\sqrt{x}}\left(\frac{\alpha^{2}-1}{\alpha^{2}}\right)$
where $\mathrm{x}=$
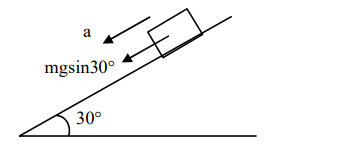
On smooth incline
$\mathrm{a}=\mathrm{g} \sin 30^{\circ}$
by $S=u t+\frac{1}{2}$ at $^{2}$
$\mathrm{S}=\frac{1}{2} \frac{\mathrm{g}}{2} \mathrm{~T}^{2}=\frac{\mathrm{g}}{4} \mathrm{~T}^{2} \ldots \ldots . .$ (i)
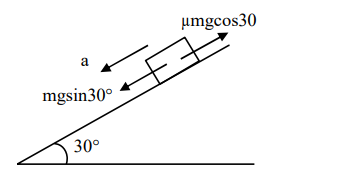
On rough incline
$\mathrm{a}=\mathrm{g} \sin 30^{\circ}-\mu \mathrm{g} \cos 30^{\circ}$
by $\mathrm{S}=\mathrm{ut}+\frac{1}{2} \mathrm{at}^{2}$
$\mathrm{S}=\frac{1}{4} \mathrm{~g}(1-\sqrt{3} \mu)(\alpha \mathrm{T})^{2} \ldots$ (ii)
By (i) and (ii)
$\frac{1}{4} \mathrm{gT}^{2}=\frac{1}{4} \mathrm{~g}(1-\sqrt{3} \mu) \alpha^{2} \mathrm{~T}^{2}$
$\Rightarrow 1-\sqrt{3} g=\frac{1}{\alpha^{2}} \Rightarrow g=\left(\frac{\alpha^{2}-1}{\alpha^{2}}\right) \cdot \frac{1}{\sqrt{3}}$
$\Rightarrow x=3.00$