Special Points of Oblique Projectile Motion :
(1) The three basic equations of motion, i.e.
$v=u+a t$ $s=u t+\frac{1}{2} a t^{2}$ $v^{2}=u^{2}+2 a s$
For projectile motion give :
$\mathrm{T}=\frac{2 \mathrm{u} \sin \theta}{\mathrm{g}}$ $R=\frac{u^{2} \sin 2 \theta}{g}$ $H=\frac{u^{2} \sin ^{2} \theta}{2 g}$
(2) In the case of projectile motion,
The horizontal component of velocity $(u \cos \theta)$, acceleration $(g)$ and mechanical energy remains constant.
Speed, velocity, the vertical component of velocity (u $\sin \theta$ ), momentum, kinetic energy, and potential energy all change. Velocity and K.E. are maximum at the point of projection, while minimum (but not zero) at the highest point.
(3) If angle of projection is changed from
$\theta \stackrel{\text { to }}{\longrightarrow} \theta^{\prime}=(90-\theta)$
then range $\quad R^{\prime}=\frac{u^{2} \sin 2 \theta^{\prime}}{g}=\frac{u^{2} \sin 2(90-\theta)}{g}=\frac{u^{2} \sin 2 \theta}{g}=R$
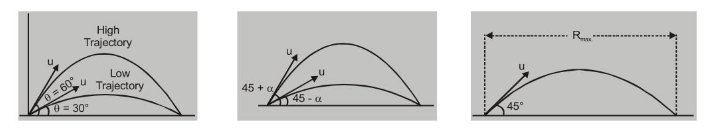
So a projectile has same range for angles of projection $\theta$ and $(90-\theta)$
But has different time of flight $(\mathrm{T})$, maximum height $(\mathrm{H}) \&$ trajectories
Range is also same for $\theta_{1}=45^{\circ}-\alpha \quad$ and $\quad \theta_{2}=45^{\circ}+\alpha .\left[\right.$ equal $\left.\frac{u^{2} \cos 2 \alpha}{g}\right]$
(4) For maximum Range
$R=R_{\max } \Rightarrow 2 \theta=90^{\circ}$
for $\quad \theta=45^{\circ}$
$R_{\max }=\frac{u^{2}}{g}$ [For $\sin 2 \theta=1=\sin 90^{\circ}$ or $\theta=45^{\circ}$ ]
When range is maximum $\Rightarrow$ Then maximum height reached
$\mathrm{H}=\frac{\mathrm{u}^{2} \sin ^{2} 45}{2 \mathrm{~g}}\left(\right.$ When $\left.\mathrm{R}_{\max }\right) \quad$ or $\quad \mathrm{H}=\frac{\mathrm{u}^{2}}{4 \mathrm{~g}}$
hence maximum height reached (for $R_{\max }$ ) $\quad H=\frac{R_{\max }}{4}$
(5) For height $\mathrm{H}$ to be maximum
$\mathrm{H}=\frac{\mathrm{u}^{2} \sin ^{2} \theta}{2 \mathrm{~g}}=\max \quad$ i.e. $\sin ^{2} \theta=1(\max )$ or for $\theta=90^{\circ}$
So that $\mathrm{H}_{\max }=\frac{\mathrm{u}^{2}}{2 \mathrm{~g}} \quad$ When projected vertically (i.e. at $\theta=90^{\circ}$ )
in this case Range $R=\frac{u^{2} \sin \left(2 \times 90^{\circ}\right)}{g}=\frac{u^{2} \sin 180^{\circ}}{g}=0$
$\mathrm{H}_{\max }=\frac{\mathrm{u}^{2}}{2 \mathrm{~g}}$ (For vertical projection) and $\mathrm{R}_{\max }=\frac{\mathrm{u}^{2}}{\mathrm{~g}}$ (For oblique projection with same velocity)
so $\quad \mathrm{H}_{\max }=\frac{\mathrm{R}_{\max }}{2}$
If a person can throw a projectile to a maximum distance $\left(w i t h, \theta=45^{\circ}\right) R_{\max }=\frac{u^{2}}{g}$.
The maximum height to which he can throw the projectile (with $\theta=90^{\circ}$ ) $\mathrm{H}_{\max }=\frac{\mathrm{R}_{\max }}{2}$
(6) At highest point
Potential energy will be max and equal to $(\mathrm{PE})_{\mathrm{H}}=\mathrm{mgH}=\mathrm{mg} \cdot \frac{\mathrm{u}^{2} \sin ^{2} \theta}{2 \mathrm{~g}}$ or $(\mathrm{PE})_{\mathrm{H}}=\frac{1}{2} \mathrm{mu}^{2} \sin ^{2} \theta$.
While K.E. will be minimum (but not zero) and at the highest point as the vertical component of velocity is zero.
$(\mathrm{KE})_{\mathrm{H}}=\frac{1}{2} \mathrm{mv}_{\mathrm{H}}^{2}=\frac{1}{2} \mathrm{~m}(\mathrm{u} \cos \theta)^{2} \quad=\frac{1}{2} \mathrm{mu}^{2} \cos ^{2} \theta$
so $(\mathrm{PE})_{\mathrm{H}}+(\mathrm{KE})_{\mathrm{H}}=\frac{1}{2} \mathrm{mu}^{2} \sin ^{2} \theta+\frac{1}{2} \mathrm{mu}^{2} \cos ^{2} \theta=\frac{1}{2} \mathrm{mu}^{2}=$ Total M.E.
So in projectile motion mechanical energy is conserved.
$\left(\frac{P E}{K E}\right)_{H}=\frac{\frac{1}{2} m u^{2} \sin ^{2} \theta}{\frac{1}{2} m u^{2} \cos ^{2} \theta}=\tan ^{2} \theta$
(7) In case of projectile motion if range $R$ is $n$ times the maximum height $H$, i.e. $R=n H$
then $\frac{u^{2} \sin 2 \theta}{g}=n \cdot \frac{u^{2} \sin ^{2} \theta}{2 g}$
or $\quad 2 \cos \theta=\frac{n \cdot \sin \theta}{2}$
or $\quad \tan \theta=\frac{4}{n} \quad \Rightarrow \quad \theta=\tan ^{-1}\left(\frac{4}{n}\right)$
(8) Weight of a body in projectile motion is zero as it is a freely falling body.
Also Read
JEE Physics Notes
To watch Free Learning Videos on JEE by Kota’s top IITian Faculties Install the eSaral App