Question:
A ray of light coming from the point $(2,2 \sqrt{3})$
is incident at an angle $30^{\circ}$ on the line $x=1$ at the point A. The ray gets reflected on the line $x=1$ and meets $x$-axis at the point $B$. Then, the line $\mathrm{AB}$ passes through the point:
Correct Option: , 2
Solution:
For point $\mathrm{A}$
$\tan 60^{\circ}=\frac{2 \sqrt{3}-\mathrm{k}}{2-1}$
$\sqrt{3}=2 \sqrt{3}-\mathrm{k}$
$\therefore \mathrm{k}=\sqrt{3}$
so point $\mathrm{A}(1, \sqrt{3})$
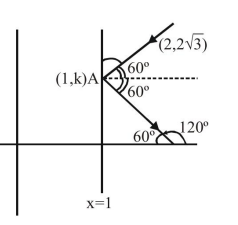
Now slope of line $\mathrm{AB}$ is $\mathrm{m}_{\mathrm{AB}}=\tan 120^{\circ}$
$\mathrm{m} \mathrm{m}_{\mathrm{AB}}=-\sqrt{3}$
Now equation of line $A B$ is
$y-\sqrt{3}=-\sqrt{3}(x-1)$
$\sqrt{3} x+y=2 \sqrt{3}$
Now satisfy options
