A square $\mathrm{ABCD}$ has all its vertices on the curve $\mathrm{x}^{2} \mathrm{y}^{2}=1$. The midpoints of its sides also lie on the same curve. Then, the square of area of $\mathrm{ABCD}$ is
$x y=1,-1$
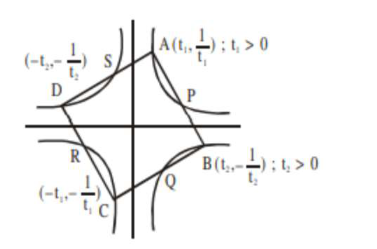
$\frac{\mathrm{t}_{1}+\mathrm{t}_{2}}{2} \cdot \frac{\frac{1}{\mathrm{t}_{1}}-\frac{1}{\mathrm{t}_{2}}}{2}=1$
$\Rightarrow \mathrm{t}_{1}^{2}-\mathrm{t}_{2}^{2}=4 \mathrm{t}_{1} \mathrm{t}_{2}$
$\frac{1}{t_{1}^{2}} \times\left(-\frac{1}{t_{2}^{2}}\right)=-1 \Rightarrow t_{1} t_{2}=1$
$\Rightarrow\left(\mathrm{t}_{1} \mathrm{t}_{2}\right)^{2}=1 \Rightarrow \mathrm{t}_{1} \mathrm{t}_{2}=1$
$\mathrm{t}_{1}^{2}-\mathrm{t}_{2}^{2}=4$
$\Rightarrow \mathrm{t}_{1}^{2}+\mathrm{t}_{2}^{2}=\sqrt{4^{2}+4}=2 \sqrt{5}$
$\Rightarrow \mathrm{t}_{1}^{2}=2+\sqrt{5} \Rightarrow \frac{1}{\mathrm{t}_{1}^{2}}=\sqrt{5}-2$
$\mathrm{AB}^{2}=\left(\mathrm{t}_{1}-\mathrm{t}_{2}\right)^{2}+\left(\frac{1}{\mathrm{t}_{1}}+\frac{1}{\mathrm{t}_{2}}\right)^{2}$
$=2\left(\mathrm{t}_{1}^{2}+\frac{1}{\mathrm{t}_{1}^{2}}\right)=4 \sqrt{5} \Rightarrow$ Area $^{2}=80$
