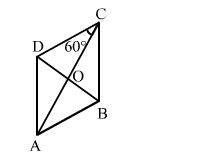
ABCD is a rhombus in which ∠C = 60°.

(a) $\sqrt{3}: 1$

ABCD is a rhombus. So all of its sides are equal.
Now, BC = DC 
⇒ ∠BDC = ∠DBC = xo   (Angles opposite to equal sides are equal)
Also, ∠BCD = 60o
∴ xo + xo + 60o = 180o 
⇒​ 2xo = 120o
⇒​ xo = 60o
i.e., ∠BCD = ∠BDC = ∠DBC =  60o
So, ​∆BCD is an equilateral triangle.
∴ BD = BC = a
Also, OB = a /2
Now, in ∆OAB, we have:

$A B^{2}=O A^{2}+O B^{2}$

$\Rightarrow O A^{2}=A B^{2}-O B^{2}=a^{2}-\left(\frac{a}{2}\right)^{2}=a^{2}-\frac{a^{2}}{4}=\frac{3 a^{2}}{4}$

$\Rightarrow O A^{2}=\frac{3 a^{2}}{4}$

$\Rightarrow O A=\sqrt{\frac{3 a^{2}}{4}}$

$\Rightarrow O A=\frac{\sqrt{3} a}{2}$

Now, $A C=2 \times O A=2 \times \frac{\sqrt{3} a}{2}=\sqrt{3} a \therefore A C: B D=\sqrt{3} a: a=\sqrt{3}: 1$