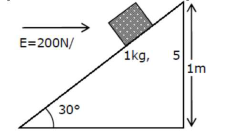
An inclined plane making an angle of $30^{\circ}$ with horizontal is placed in a uniform horizontal electric field $200 \frac{N}{C}$ as shown in the figure. A body of mass $1 \mathrm{~kg}$ and charge $5 \mathrm{mC}$ is allowed to slide down from rest at a height of $1 \mathrm{~m}$. If the coefficient of frication is $0.2$, find the time taken by the body to reach the bottom.
$\left[g=9.8 \mathrm{~m} / \mathrm{s}^{2}, \sin 30^{\circ}=\frac{1}{2} ; \cos 30^{\circ}=\frac{\sqrt{3}}{2}\right]$
Correct Option: , 3
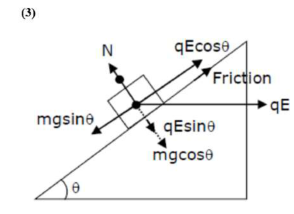
$F=m g \sin \theta-(\mu N+q E \cos \theta)$
$F=m g \sin \theta-\mu(m g \cos \theta+q E \sin \theta)-q E \cos \theta$
$F=1 \times 10 \times \sin 30-0.2\left(1 \times 10 \times \cos 30^{\circ}+200 \times 5 \times 10^{-3} \sin 30^{\circ}\right)$
$-200 \times 5 \times 10^{-3} \cos 30^{\circ}$
$F=2.3 \mathrm{~N}$
\begin{aligned}
&a=\frac{F}{m} \Rightarrow \frac{2.3}{1} \Rightarrow 2.3 \mathrm{~m} / \mathrm{sec}^{2} \\
&\mathrm{t}=\sqrt{\frac{25}{9}} \Rightarrow \sqrt{\frac{2 \times 2}{2.3}} \Rightarrow 1.3 \mathrm{sec}
\end{aligned}
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.