Question:
$B D$ is one of the diagonals of a quad. $A B C D$. If $A L \perp B D$ and $C M \perp B D$, show that ar (quad. $A B C D$ ) $=\frac{1}{2} \times B D \times(A L+C M)$.
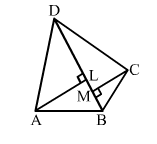
Solution:
ar(quad. ABCD) = ar(∆ABD) + ar (∆DBC)
$\operatorname{ar}(\Delta A B D)=\frac{1}{2} \times$ base $\times$ height $=\frac{1}{2} \times B D \times A L$ ...(i)
$\operatorname{ar}(\Delta D B C)=\frac{1}{2} \times B D \times C L$ ...(ii)
From (i) and (ii), we get:
$\operatorname{ar}($ quad $A B C D)=\frac{1}{2} \times B D \times A L+\frac{1}{2} \times B D \times C L$
$\Rightarrow \operatorname{ar}($ quad $A B C D)=\frac{1}{2} \times B D \times(A L+C L)$
Hence, proved.
