Consider the parabola with vertex $\left(\frac{1}{2}, \frac{3}{4}\right)$ and the $\operatorname{directrix} y=\frac{1}{2} .$ Let $P$ be the point where the parabola meets the line $x=-\frac{1}{2}$. If the normal to the parabola at $\mathrm{P}$ intersects the parabola again at the point $Q$, then $(P Q)^{2}$ is equal to:
Correct Option: , 2
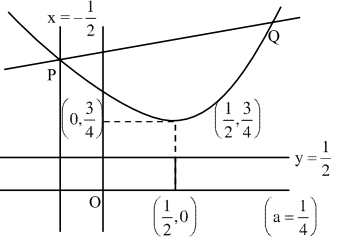
$\left(y-\frac{3}{4}\right)=\left(x-\frac{1}{2}\right)^{2}$
For $x=-\frac{1}{2}$
$y-\frac{3}{4}=1 \Rightarrow y=\frac{7}{4} \Rightarrow P\left(-\frac{1}{2}, \frac{7}{4}\right)$
Now $\mathrm{y}^{\prime}=2\left(\mathrm{x}-\frac{1}{2}\right) \quad$ At $\mathrm{x}=-\frac{1}{2}$
$\Rightarrow \mathrm{m}_{\mathrm{T}}=-2, \mathrm{~m}_{\mathrm{N}}=\frac{1}{2}$
Equation of Normal is
$\mathrm{y}-\frac{7}{4}=\frac{1}{2}\left(\mathrm{x}+\frac{1}{2}\right)$
$y=\frac{x}{2}+2$
Now put y in equation (1)
$\frac{x}{2}+2-\frac{3}{4}=\left(x-\frac{1}{2}\right)^{2}$
$\Rightarrow \mathrm{x}=2 \&-\frac{1}{2}$
$\Rightarrow \mathrm{Q}(2,3)$
Now $(\mathrm{PQ})^{2}=\frac{125}{16}$
Option (2)
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
