Draw the graphs of the lines 2x + y = 6 and 2x – y + 2 = 0. Shade the region bounded by these lines and the x-axis.
The lines 2x + y = 6 and 2x – y + 2 = 0 form a triangle with the x-axis, and the area of the shaded region is 8 square units.
Draw the graphs of the lines 2x + y = 6 and 2x – y + 2 = 0. Shade the region bounded by these lines and the x-axis. Find the area of the shaded region.
$2 x+y=6$
$\Rightarrow y=-2 x+6$
When $x=0, y=-2 \times 0+6=0+6=6$
When $x=1, y=-2 \times 1+6=-2+6=4$
When $x=2, y=-2 \times 2+6=-4+6=2$
Thus, the points on the line 2x + y = 6 are as given in the following table:
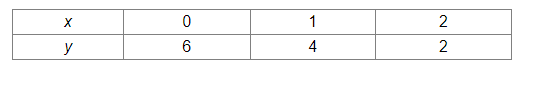
Plotting the points (0, 6), (1, 4) and (2, 2) and drawing a line passing through these points, we obtain the graph of of the line 2x + y = 6.
$2 x-y+2=0$
$\Rightarrow y=2 x+2$
When $x=0, y=2 \times 0+2=0+2=2$
When $x=1, y=2 \times 1+2=2+2=4$
When $x=-1, y=2 \times(-1)+2=-2+2=0$
Thus, the points on the line 2x – y + 2 = 0 are as given in the following table:
Plotting the points (0, 2), (1, 4) and (–1, 0) and drawing a line passing through these points, we obtain the graph of of the line 2x – y + 2 = 0.
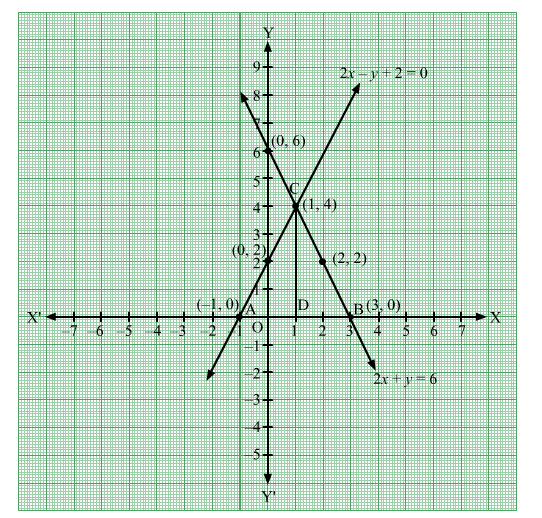
The shaded region represents the area bounded by the lines 2x + y = 6, 2x – y + 2 = 0 and the x-axis. This represents a triangle.
It can be seen that the lines intersect at the point C(1, 4). Draw CD perpendicular from C on the x-axis.
Height = CD = 4 units
Base = AB = 4 units
$\therefore$ Area of the shaded region = Area of $\Delta \mathrm{ABC}=\frac{1}{2} \times \mathrm{AB} \times \mathrm{CD}=\frac{1}{2} \times 4 \times 4=8$ square units
Frequently Asked Questions
Find answers to common questions.
What is the area of the region bounded by 2x + y = 6, 2x – y + 2 = 0, and the x-axis?
The area of the bounded region is 8 square units. The two lines intersect at C(1, 4), and they meet the x-axis at A(–1, 0) and B(3, 0), forming a triangle with base AB = 4 units and height CD = 4 units. Area = ½ × 4 × 4 = 8 sq. units.
Where do the lines 2x + y = 6 and 2x – y + 2 = 0 intersect?
The lines intersect at the point (1, 4). Solving simultaneously: adding both equations gives 4x + 2 = 8 — wait, let's be precise. Setting –2x + 6 = 2x + 2 gives 4x = 4, so x = 1 and y = 4. You can verify this point satisfies both original equations.
How do you find the x-intercept of 2x + y = 6?
To find the x-intercept, substitute y = 0 into the equation: 2x + 0 = 6, giving x = 3. So the x-intercept is (3, 0). This is one vertex of the triangle bounded by the two lines and the x-axis.
How do you find the x-intercept of 2x – y + 2 = 0?
Substitute y = 0: 2x – 0 + 2 = 0, giving 2x = –2, so x = –1. The x-intercept is (–1, 0). This is the second vertex of the triangle on the x-axis. The base of the triangle runs from x = –1 to x = 3, a length of 4 units.
What shape is formed by 2x + y = 6, 2x – y + 2 = 0, and the x-axis?
The three boundaries — line 1, line 2, and the x-axis — form a triangle. The three vertices are A(–1, 0), B(3, 0), and C(1, 4). Since the base lies flat on the x-axis, calculating the area is straightforward using Area = ½ × base × height.