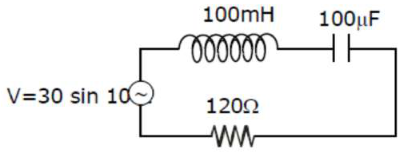
Find the peak current and resonant frequency of the following circuit (as shown in figure)

(4)

Peak current in series LCR CKT

$i=\frac{v_{0}}{z} \Rightarrow \frac{30}{\sqrt{\left(x_{L}-x_{C}\right)^{2}+R^{2}}}$

$i=\frac{30}{\sqrt{(10-100)^{2}+(120)^{2}}}$

$i \Rightarrow \frac{30}{150} \Rightarrow \frac{1}{5} \Rightarrow 0.2 \mathrm{Amp}$

$\therefore X_{L}=\omega \times L$

$\Rightarrow(100)\left(100 \times 10^{-3}\right) \Rightarrow 10$

$X_{L}=\frac{1}{\omega \times c} \Rightarrow \frac{1}{100 \times 100 \times 10^{-6}}$

$\Rightarrow \frac{10^{6}}{10^{4}} \Rightarrow 100$

Resonance frequency $\omega=\frac{1}{\sqrt{L C}}$

$\omega=\frac{1}{\sqrt{100 \times 10^{-3} \times 100 \times 10^{-6}}} \Rightarrow \frac{1}{\sqrt{10^{-5}}}$

$\therefore \omega=2 \pi F$

$F=\frac{1}{2 \pi} \times \frac{1}{\sqrt{10^{-5}}}$

$\Rightarrow \frac{1}{2 \pi} \sqrt{10^{5}}$

$\Rightarrow \frac{100}{2 \pi} \sqrt{10}$

$\Rightarrow 50 \mathrm{~Hz}$