Question:
For the given cell;
$\mathrm{Cu}(\mathrm{s})\left|\mathrm{Cu}^{2+}\left(\mathrm{C}_{1} \mathrm{M}\right)\right|\left|\mathrm{Cu}^{2+}\left(\mathrm{C}_{2} \mathrm{M}\right)\right| \mathrm{Cu}(\mathrm{s})$
change in Gibbs energy $(\Delta \mathrm{G})$ is negative, if:
Correct Option: , 4
Solution:
For the concentration cell, $E_{\text {cell }}^{0}=0$
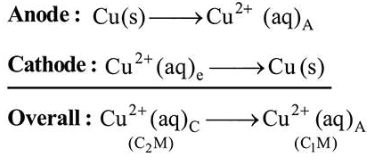
As $\Delta G=-n F E$
If $\Delta G=-\mathrm{ve}$, then $E_{\text {cell }}$ is $+\mathrm{v}_{\mathrm{e}}$.
$E_{\text {cell }}=E_{\text {cell }}^{\mathrm{o}}-\frac{R T}{2 F} \ln \frac{C_{1}}{C_{2}}$
$E_{\text {cell }}=0-\frac{R T}{2 F} \ln \frac{C_{1}}{C_{2}}$
$E_{\text {cell }}=\frac{R T}{2 F} \ln \frac{C_{2}}{C_{1}}$
So, $C_{2}>C_{1}$
Thus, $C_{2}=\sqrt{2} C_{1}$ relation is correct.
