If A ⊂ B, show that (B’ – A’) = ϕ.

As $A \subset B$ the set $A$ is inside set $B$

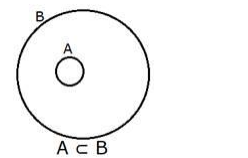
Hence $A \cup B=B$

Taking compliment

$\Rightarrow(\mathrm{A} \cup \mathrm{B})^{\prime}=\mathrm{B}^{\prime}$

Using De-Morgan's law $(A \cup B)^{\prime}=A^{\prime} \cap B^{\prime}$

$\Rightarrow A^{\prime} \cap B^{\prime}=B^{\prime} \ldots$ (i)

Now we know that

$B^{\prime}=\left(B^{\prime}-A^{\prime}\right)+\left(A^{\prime} \cap B^{\prime}\right)$

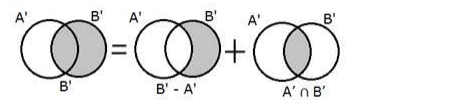
Using (i)

$\Rightarrow B^{\prime}=\left(B^{\prime}-A^{\prime}\right)+B^{\prime}$

$\Rightarrow\left(B^{\prime}-A^{\prime}\right)=B^{\prime}-B^{\prime}$

$\Rightarrow\left(B^{\prime}-A^{\prime}\right)=0$

$\Rightarrow\left(B^{\prime}-A^{\prime}\right)=\{\phi\}$

Hence proved