Question:
If O is a point within a quadrilateral ABCD, show that OA + OB + OC + OD > AC + BD.
Solution:
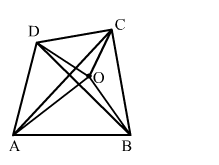
Let ABCD be a quadrilateral whose diagonals are AC and BD and O is any point within the quadrilateral.
Join O with A, B, C, and D.
We know that the sum of any two sides of a triangle is greater than the third side.
So, in ∆AOC, OA + OC > AC
Also, in ∆ BOD, OB + OD > BD
Adding these inequalities, we get:
(OA + OC) + (OB + OD) > (AC + BD)
⇒ OA + OB + OC + OD > AC + BD
⇒ OA + OB + OC + OD > AC + BD
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.