Question:
If the function $f$ given by $f(x)=x^{3}-3(a-2) x^{2}+3 a x+7$, for some $\mathrm{a} \in \mathrm{R}$ is increasing in $(0,1]$ and decreasing in $[1,5)$, then a root of the equation,
$\frac{f(x)-14}{(x-1)^{2}}=0(x \neq 1)$ is
Correct Option: , 3
Solution:
$f(x)=x^{3}-3(a-2) x^{2}+3 a x+7, f(0)=7$
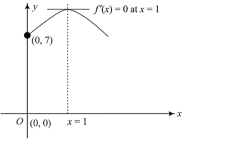
$\Rightarrow \quad f^{\prime}(x)=3 x^{2}-6(a-2) x+3 a$
$f^{\prime}(1)=0$
$\Rightarrow \quad 1-2 a+4+a=0$
$\Rightarrow \quad a=5$
Then, $f(x)=x^{3}-9 x^{2}+15 x+7$
Now,
$\frac{f(x)-14}{(x-1)^{2}}=0$
$\Rightarrow \quad \frac{x^{3}-9 x^{2}+15 x+7-14}{(x-1)^{2}}=0$
$\Rightarrow \quad \frac{(x-1)^{2}(x-7)}{(x-1)^{2}}=0 \Rightarrow x=7$
