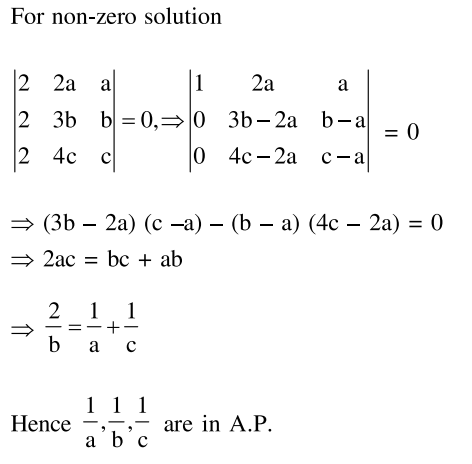
Question:
If the system of linear equations
$2 x+2 a y+a z=0$
$2 x+3 b y+b z=0$
$2 x+4 c y+c z=0$
where $a, b, c \in R$ are non-zero and distinct; has a non-zero solution, then :
Correct Option: , 4
Solution:
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
