In a quadrilateral ABCD, show that

Solution:

Given: Quadrilateral ABCD
To prove: (AB + BC + CD + DA) < 2(BD + AC).
Proof:

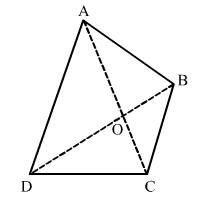
In ∆AOB, 

$O A+O B>A B \quad \ldots \ldots(i)$

In ∆BOC, 

$O B+O C>B C \quad \ldots \ldots(i i)$

In ∆COD, 

$O C+O D>C D \quad \ldots \ldots($ iii $)$

In ∆AOD, 

$O D+O A>A D \quad \ldots \ldots(i v)$

Adding $(i),(i i),(i i i)$ and $(i v)$, we get

$2(O A+O B+O C+O D)>(A B+B C+C D+D A)$

$\Rightarrow 2(O B+O D+O A+O C)>(A B+B C+C D+D A)$

$\Rightarrow 2(B D+A C)>(A B+B C+C D+D A)$