In a Young's double slit experiment, the width of the one of the slit is three times the other slit. The amplitude of the light coming from a slit is proportional to the slit-width. Find the ratio of the maximum to the minimum intensity in the
Correct Option: 1
(1)
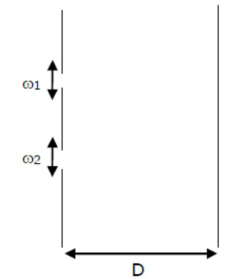
given $: \omega_{2}=3 \omega_{1}$
also, $A \propto \omega$
$\frac{\omega_{1}}{\omega_{2}}=\frac{1}{3} \cdots(1)$
Assume $\omega_{1}=x, \omega_{2}=3 x$
we know that
$I_{\max }=\left(A_{1}+A_{2}\right)^{2}$, and
$I_{\min }=\left(A_{1}-A_{2}\right)^{2}$
$\frac{A_{1}}{A_{2}}=\frac{\omega_{1}}{\omega_{2}} \ldots(2)$
from equation (2) we can say that
$A_{1}=A$ and $A_{2}=3 A$
Now, $\frac{I_{\max }}{I_{\min }}=\frac{(A+3 A)^{2}}{(A-3 A)^{2}}=\frac{16 A^{2}}{4 A^{2}}=\frac{4}{1}$
$\Rightarrow \frac{I_{\max }}{I_{\min }}=\frac{4}{1}$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.