In ∆ABC, side AB is produced to D such that BD = BC. If ∠B = 60° and ∠A = 70°,

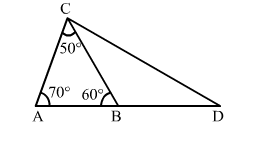
In triangle CBA, CBD is an exterior angle.

i. e., $\angle C B A+\angle C B D=180^{\circ}$

$\Rightarrow 60^{\circ}+\angle C B D=180^{\circ}$

$\Rightarrow \angle C B D=120^{\circ}$

Triangle BCD is isosceles and BC = BD.

Let $\angle B C D=\angle B D C=x^{\circ}$.

In $\triangle C B D$, we have :

$\Rightarrow \angle B C D+\angle C B D+\angle C D B=180^{\circ}$

$\Rightarrow x+120^{\circ}+x=180$

$\Rightarrow 2 x=60^{\circ}$

$\Rightarrow x=30^{\circ}$

$\therefore \angle B C D=\angle B D C=30^{\circ}$

In triangle ADC, $\angle C=\angle A C B+\angle B C D=50^{\circ}+30^{\circ}=80^{\circ}$

$\angle A=70^{\circ}$

and $\angle D=30^{\circ}$

$\therefore \angle C>\angle A$

$\Rightarrow A D>C D \quad \ldots(1)$

Also, $\angle C>\angle D$

$\Rightarrow A D>A C \quad \ldots(2)$