Question:
In an equilateral triangle ABC, if AD ⊥ BC, then which of the following is true?
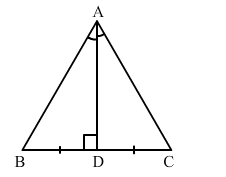
(a) 2AB2 = 3AD2
(b) 4AB2 = 3AD2
(c) 3AB2 = 4AD2
(d) 3AB2 = 2AD2
Solution:
(c) 3AB2 = 4AD2
Applying Pythagoras theorem in right-angled triangles ABD and ADC, we get:
$A B^{2}=A D^{2}+B D^{2}$
$\Rightarrow A B^{2}=\left(\frac{1}{2} A B\right)^{2}+A D^{2} \quad\left(\because \triangle \mathrm{ABC}\right.$ is equilateral and $\left.A D=\frac{1}{2} \mathrm{AB}\right)$
$\Rightarrow A B^{2}=\frac{1}{4} A B^{2}+A D^{2}$
$\Rightarrow A B^{2}-\frac{1}{4} A B^{2}=A D^{2}$
$\Rightarrow \frac{3}{4} A B^{2}=A D^{2}$
$\Rightarrow 3 A B^{2}=4 A D^{2}$
