In an experiment, the resistance of a material is plotted as a function of temperature (in some range).
Question:
In an experiment, the resistance of a material is plotted as a function of temperature (in some range). As shown in the figure, it is a straight line.
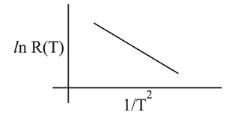
One may canclude that:
Correct Option: 2,
Solution:
(2) Equation of straight line from graph
$\mathrm{y}=-\mathrm{mx}+\mathrm{c}$
$\Rightarrow l \mathrm{nR}=-\mathrm{m}\left(\frac{1}{\mathrm{~T}^{2}}\right)+\mathrm{c}$
here, $m$ & $c$ are constants
$\mathrm{R}=\mathrm{e}^{\left[-\mathrm{m}\left(\frac{1}{\mathrm{~T}^{2}}\right)+\mathrm{c}\right]}$
$=e^{-m\left(\frac{1}{T^{2}}\right)} \times e^{c}$
$R(T)=R_{0} e^{\frac{-T_{0}^{2}}{T^{2}}}$
