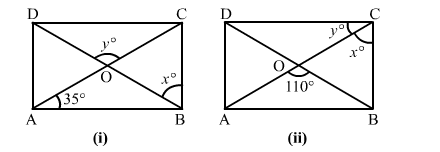
In each of the figures given below, ABD is a rectangle.

(i) ABCD is a rectangle.
The diagonals of a rectangle are congruent and bisect each other. Therefore, in​ ∆ AOB, we have:
   OA = OB   
 ∴​ ∠​OAB = ∠​OBA = 35o
∴​ x = 90o − 35o = 55o
And ∠AOB = 180o − (35o + 35o) = 110o
∴​ y = ∠AOB​ = 110o​                     [Vertically opposite angles]
Hence, x = 55o and y = 110o​​

(ii) In ∆AOB, we have:
      OA = OB   

Now, $\angle O A B=\angle O B A=\frac{1}{2} \times\left(180^{\circ}-110^{\circ}\right)=35^{\circ}$

∴​ y = ∠BAC = 35o                 [Interior alternate angles]
Also, x = 90o − y                          [ ​∵∠C = 90o =  x + y ]
⇒​ x = 90o − 35o = 55o                     
Hence, x = 55o and y = 35o