In figure, $\mathrm{DE} \| \mathrm{AC}$ and $\mathrm{DF} \| \mathrm{AE}$. Prove that $\frac{\mathrm{BF}}{\mathrm{FE}}=\frac{\mathrm{BE}}{\mathrm{EC}}$.
Question.
In figure, $D E \| A C$ and $D F \| A E$. Prove that $\frac{B F}{F E}=\frac{B E}{E C}$.
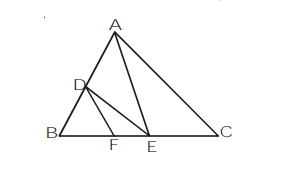
In figure, $D E \| A C$ and $D F \| A E$. Prove that $\frac{B F}{F E}=\frac{B E}{E C}$.

Solution:
In $\triangle \mathrm{ABE}$
$\mathrm{DF} \| \mathrm{AE}$ (Given)
$\frac{B D}{D A}=\frac{B F}{F E} \ldots$ (i) (Basic Proportionality Theorem)
In $\triangle \mathrm{ABC}$,
$D E \| A C$(Given)
$\frac{B D}{D A}=\frac{B E}{E C}$ (ii) (Basic Proportionality Theorem)
From (i) and (ii), we get
$\frac{B F}{F E}=\frac{B E}{E C} \quad$ Hence proved.
In $\triangle \mathrm{ABE}$
$\mathrm{DF} \| \mathrm{AE}$ (Given)
$\frac{B D}{D A}=\frac{B F}{F E} \ldots$ (i) (Basic Proportionality Theorem)
In $\triangle \mathrm{ABC}$,
$D E \| A C$(Given)
$\frac{B D}{D A}=\frac{B E}{E C}$ (ii) (Basic Proportionality Theorem)
From (i) and (ii), we get
$\frac{B F}{F E}=\frac{B E}{E C} \quad$ Hence proved.
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
