Question:
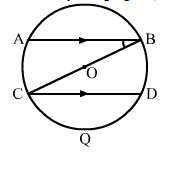
In the adjoining figure, BC is a diameter of a circle with centre O. If AB and CD are two chords such that AB || CD, prove that AB = CD.
Solution:
Given: BC is a diameter of a circle with centre O. AB and CD are two chords such that AB || CD.
TO prove: AB = CD
Construction: Draw OL ⊥ AB and OM ⊥ CD.
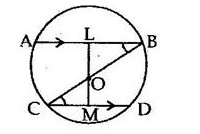
Proof:
In ΔOLB and ΔOMC, we have:
∠OLB = ∠OMC [90° each]
∠OBL = ∠OCD [Alternate angles as AB || CD]
OB = OC [Radii of a circle]
∴ ΔOLB ≅ ΔOMC (AAS criterion)
Thus, OL = OM (CPCT)
We know that chords equidistant from the centre are equal.
Hence, AB = CD
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.