In the adjoining figure, MNPQ and ABPQ are parallelograms and T is any point on the side BP. Prove that

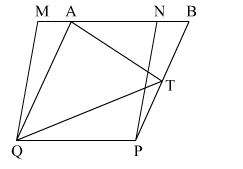
(i) We know that parallelograms on the same base and between the same parallels are equal in area.
So, ar(MNPQ) = are(ABPQ)                   (Same base PQ and MB || PQ)                      .....(1)

(ii) If a parallelogram and a triangle are on the same base and between the same parallels then the area of the triangle is equal to half the area of the parallelogram. 

So, $\operatorname{ar}(\Delta A T Q)=\frac{1}{2} \operatorname{ar}(A B P Q)$      (Same base $A Q$ and $A Q \| B P$ )               .....(2)

From (1) and (2)

$\operatorname{ar}(\Delta A T Q)=\frac{1}{2} \operatorname{ar}(M N P Q)$