Question:
In the given figure, a || gm ABCD and a rectangle ABEF are of equal area. Then,
(a) perimeter of ABCD = perimeter of ABEF
(b) perimeter of ABCD < perimeter of ABEF
(c) perimeter of ABCD > perimeter of ABEF
(d) perimeter of $A B C D=\frac{1}{2}$ (perimeter of $A B E F$ )
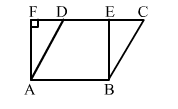
Solution:
(c) perimeter of ABCD > perimeter of ABEF
Parallelogram ABCD and rectangle ABEF lie on the same base AB, i.e., one side is common in both the figures.
In ||gm ABCD, we have:
AD is the hypotenuse of right angled triangle ADF.
So, AD > AF
∴ Perimeter of ABCD > perimeter of ABEF
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.