Question:
In the given figure, AB || CD. If ∠EAB = 50° and ∠ECD = 60°, then ∠AEB = ?
(a) 50°
(b) 60°
(c) 70°
(d) 55°
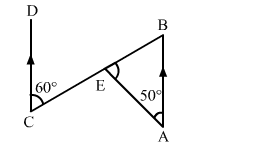
Solution:
(c) 70°
$A B \| C D$ and $B C$ is the transversal.
$\therefore \angle A B E=\angle B C D=60^{\circ} \quad[$ Alternate Internal Angles $]$
In $\Delta A B E$, we have:
$\angle E A B+\angle A B E+\angle A E B=180^{\circ} \quad[$ Sum of the angles of a triangle $]$
$\Rightarrow 50^{\circ}+60^{\circ}+\angle A E B=180^{\circ}$
$\Rightarrow \angle A E B=70^{\circ}$
