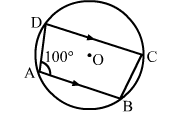
Question:
In the given figure ABCD is a cyclic quadrilateral in which AB || DC and ∠BAD = 100°. Then, ∠ABC = ?
(a) 80°
(b) 100°
(c) 50°
(d) 40°
Solution:
(b) 100°
Since ABCD is a cyclic quadrilateral, we have:
∠BAD + ∠BCD = 180° (Opposite angles of a cyclic quadrilateral)
⇒ 100° + ∠BCD = 180°
⇒ ∠BCD = (180° - 100°) = 80°
Now, AB || DC and CB is the transversal.
∴ ∠ABC + ∠BCD = 180°
⇒ ∠ABC + 80° = 180°
⇒ ∠ABC = (180° - 80°) = 100°
