In the given figure, ABCD is a parallelogram, M is the mid-point of BD and BD bisects ∠B as well as ∠D.
Question:
In the given figure, ABCD is a parallelogram, M is the mid-point of BD and BD bisects ∠B as well as ∠D. Then, ∠AMB = ?
(a) 45°
(b) 60°
(c) 90°
(d) 30°
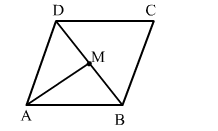
Solution:
(c) 90°
Explanation:
∠B = ∠D
$\Rightarrow \frac{1}{2} \angle B=\frac{1}{2} \angle D$
⇒ ∠ADB = ∠ABD
∴ ∆ABD is an isosceles triangle and M is the midpoint of BD. We can also say that M is the median of ∆ABD.
∴ AM ⊥ BD and, hence, ∠AMB = 90°
