In the given figure, AOB is a straight line. If ∠AOC = (3x − 10)°, ∠COD = 50° and ∠BOD = (x + 20)°, then ∠AOC = ?
Question:
In the given figure, AOB is a straight line. If ∠AOC = (3x − 10)°, ∠COD = 50° and ∠BOD = (x + 20)°, then ∠AOC = ?
(a) 40°
(b) 60°
(c) 80°
(d) 50°
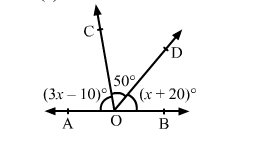
Solution:
(c) 80°
We have :
$\angle A O C+\angle C O D+\angle B O D=180^{\circ} \quad$ [Since $A O B$ is a straight line ]
$\Rightarrow 3 x-10+50+x+20=180$
$\Rightarrow 4 x=120$
$\Rightarrow x=30$
$\therefore \angle A O C=[3 \times 30-10]^{\circ}$
$\Rightarrow \angle A O C=80^{\circ}$
