Question:
In the given figure, BA || ED and BC || EF. Show that ∠ABC = ∠DEF.
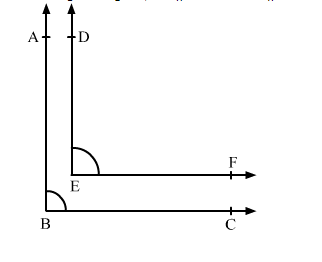
Solution:
It is given that, BA || ED and BC || EF.
Construction: Extend DE such that it intersects BC at J. Also, extend FE such that it intersects AB at H.
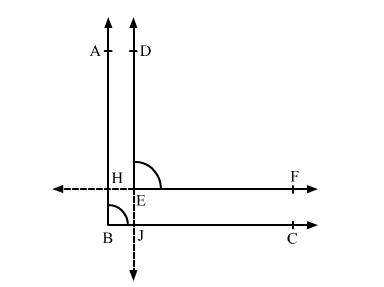
Now, BA || JD and BC is a transversal.
∴ ∠ABC = ∠DJC .....(1) (Pair of corresponding angles)
Also, BC || HF and DJ is a transversal.
∴ ∠DJC = ∠DEF .....(2) (Pair of corresponding angles)
From (1) and (2), we have
∠ABC = ∠DEF
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
