In the given figure, l || m and a transversal t cuts them.

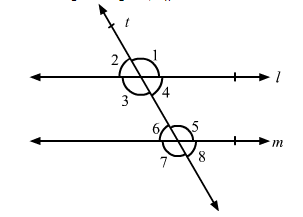
We have, $\angle 1=120^{\circ}$. Then,

$\angle 1=\angle 5$ [Corresponding angles]

$\Rightarrow \angle 5=120^{\circ}$

$\angle 1=\angle 3$ [Vertically-opposite angles]

$\Rightarrow \angle 3=120^{\circ}$

$\angle 5=\angle 7$ [Vertically-opposite angles]

$\Rightarrow \angle 7=120^{\circ}$

$\angle 1+\angle 2=180^{\circ}$ [Since AFB is a straight line]

$\Rightarrow 120^{\circ}+\angle 2=180^{\circ}$

$\Rightarrow \angle 2=60^{\circ}$

$\angle 2=\angle 4$ [Vertically-opposite angles]

$\Rightarrow \angle 4=60^{\circ}$

$\angle 2=\angle 6$ [Corresponding angles]

$\Rightarrow \angle 6=60^{\circ}$

$\angle 6=\angle 8$ [Vertically-opposite angles]

$\Rightarrow \angle 8=60^{\circ}$

$\therefore \angle 1=120^{\circ}, \angle 2=60^{\circ}, \angle 3=120^{\circ}, \angle 4=60^{\circ}, \angle 5=120^{\circ}$,

$\angle 6=60^{\circ}, \angle 7=120^{\circ}$ and $\angle 8=60^{\circ}$