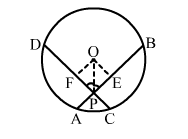
In the given figure, O is the centre of a circle in which chords AB and CD intersect at P such that PO bisects ∠BPD.

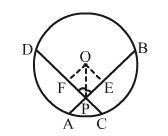
Given: O is the centre of a circle in which chords AB and CD intersect at P such that PO bisects ∠BPD.
To prove: AB = CD
Construction: Draw OE ⊥ AB and OF ⊥ CD
Proof: In ΔOEP and ΔOFP, we have:
∠OEP = ∠OFP         (90° each)
OP = OP                   (Common)
∠OPE = ∠OPF         (∵ OP bisects ∠BPD )
Thus, ΔOEP ≅ ΔOFP      (AAS criterion)
⇒ OE = OF               
Thus, chords AB and CD are equidistant from the centre O.
⇒  AB = CD         (∵ Chords equidistant from the centre are equal)
∴ AB =  CD