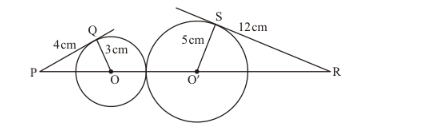
In the given figure, PR =

Solution:

We know that the radius will always be perpendicular to the tangent at the point of contact.

Therefore,

$O Q \perp O P$

Hence we have,

$O P^{2}=O Q^{2}+Q P^{2}$

$O P^{2}=4^{2}+3^{2}$

$O P^{2}=16+9$

 

$O P^{2}=25$

$O P=\sqrt{25}$

 

$O P=5$

Also,

OO’ = sum of the radii of the two circles

OO’ = 3 + 5

OO’ = 8

Since radius is perpendicular to the tangent, . Therefore,

$O^{\prime} R^{2}=O^{\prime} S^{2}+S R^{2}$

$O^{\prime} R^{2}=12^{2}+5^{2}$

$O^{\prime} R^{2}=144+25$

$O^{\prime} R^{2}=169$

$O^{\prime} R=\sqrt{169}$

$O^{\prime} R=13$

PR = PO + OO’ + O’R

PR = 5 + 8 + 13

PR =26

Therefore, option (b) is correct.