Question:
In the given figure, side BC of ∆ABC is produced to D. If ∠ACD = 128° and ∠ABC = 43°, find ∠BAC and ∠ACB.
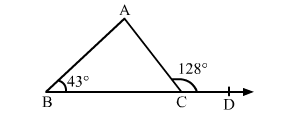
Solution:
Side BC of triangle ABC is produced to D.
$\therefore \angle A C D=\angle A+\angle B \quad$ [Exterior angle property]
$\Rightarrow 128^{\circ}=\angle A+43^{\circ}$
$\Rightarrow \angle A=(128-43)^{\circ}$
$\Rightarrow \angle A=85^{\circ}$
$\Rightarrow \angle B A C=85^{\circ}$
Also, in triangle ABC,
$\angle B A C+\angle A B C+\angle A C B=180^{\circ} \quad$ [Sum of the angles of a triangle]
$\Rightarrow 85^{\circ}+43^{\circ}+\angle A C B=180^{\circ}$
$\Rightarrow 128^{\circ}+\angle A C B=180^{\circ}$
$\Rightarrow \angle A C B=52^{\circ}$
