Question:
On one page of a telephone directory, there are 200 phone numbers. The frequency distribution of their units digits is given below:
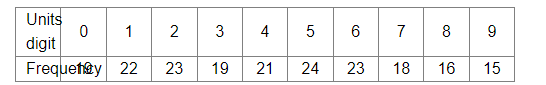
One of the numbers is chosen at random from the page. What is the probability that the units digit of the chosen number is
(i) 5?
(ii) 8?
Solution:
Total phone numbers on the directory page = 200
(i) Number of numbers with units digit 5 = 24
Let E1 be the event that the units digit of selected number is 5.
$\therefore$ Required probability $=P\left(E_{1}\right)=\frac{24}{200}=0.12$
(ii) Number of numbers with units digit 8 = 16
Let E2 be the event that the units digit of selected number is 8.
$\therefore$ Required probability $=P\left(E_{2}\right)=\frac{16}{200}=0.08$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.