Question:
Let $\alpha \in \mathrm{R}$ and the three vectors
$\overrightarrow{\mathrm{a}}=\alpha \hat{\mathrm{i}}+\hat{\mathrm{j}}+3 \hat{\mathrm{k}}, \quad \overrightarrow{\mathrm{b}}=2 \hat{\mathrm{i}}+\hat{\mathrm{j}}-\alpha \hat{\mathrm{k}} \quad$ and
$\overrightarrow{\mathrm{c}}=\alpha \hat{\mathrm{i}}-2 \hat{\mathrm{j}}+3 \hat{\mathrm{k}} \cdot$ Then the set $\mathrm{S}=\{\alpha: \overrightarrow{\mathrm{a}}, \overrightarrow{\mathrm{b}}$ and $\overrightarrow{\mathrm{c}}$ are coplanar $\}$
Correct Option: , 4
Solution:
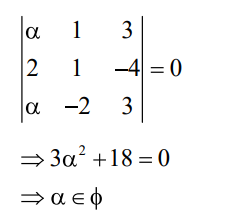
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
