Solve

$|x|>4$

Square

$\Rightarrow x^{2}>16$

$\Rightarrow x^{2}-16>0$

$\Rightarrow x^{2}-4^{2}>0$

$\Rightarrow(x+4)(x-4)>0$

Observe that when x is greater than 4, (x + 4)(x – 4) is positive

And for each root the sign changes hence

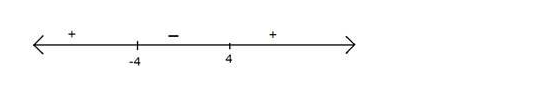
We want greater than 0 that is positive part

Hence $x$ should be less than $-4$ and greater than 4 for $(x+4)(x-4)$ to be positive

$x$ less than $-4$ means $x$ is from negative infinity to $-4$ and $x$ greater than 4 means $x$ is from 4 to infinity

Hence $x \in(-\infty,-4)$ and $x \in(4, \infty)$

Hence the solution set of $|x|>4$ is $(-\infty,-4) \cup(4, \infty)$