Question:
Find the acceleration of the block of mass $M$ in the situation of figure. The coefficient of friction between the two blocks is $\mu 1$, and that between the bigger block and the ground is $\mu 2$.
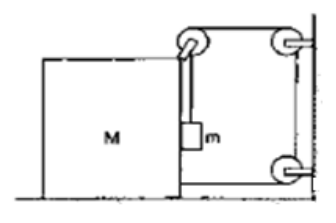
Solution:
When a block M, moves with acceleration a towards right block $\mathrm{m}$ moves downwards and rightwards with acceleration $2 a$ and a respectively.
$N^{\prime}=M g+\mu_{1} N+T$ (Vertical) -(i)
$(T+T)-N-\mu_{2} N^{\prime}=M a$ (Horizontal) -(ii)
FBD of mass $\mathrm{m}$
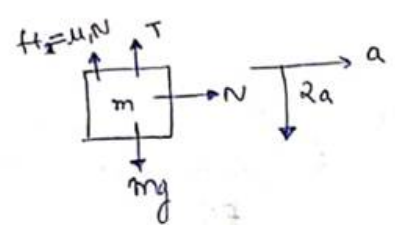
N=ma (Horizontal) -(iii)
$m g-T-\mu_{1} N=m(2 a)$ (vertical) -(iv)
From (iii) and (iv)
$T=m g-2 m a-\mu_{1} m a-(\mathrm{v})$
Solving equation (i), (ii), (iii) and (iv)
$a=\frac{\left[2 m-\mu_{2}(M+m)\right] g}{M+m\left[5+2\left(\mu_{1}-\mu_{2}\right)\right]}$
