Four particles having masses $\mathrm{m}, 2 \mathrm{~m}, 3 \mathrm{~m}$ and $4 \mathrm{~m}$ are placed at the four corners of a square of edge a. Find the gravitational force acting on a particle of mass $\mathrm{m}$ placed at the center.
$r=a^{a} / \sqrt{2}$
Here $\overrightarrow{F_{1}}=\frac{G \cdot m \cdot m}{r^{2}}$
$=\frac{\text { G.mm }}{a^{2}}(2)=2 k$
$\mathrm{K}=\frac{G m^{2}}{a^{2}}$
$\overrightarrow{F_{2}}=\frac{G \cdot 2 m \cdot m}{r^{2}}=4 \mathrm{~K}$
$\overrightarrow{F_{3}}=\frac{G \cdot 3 m \cdot m}{r^{2}}=6 K$
$\overrightarrow{F_{4}}=\frac{G .4 m \cdot m}{r^{2}}=8 \mathrm{~K}$
Here $\overrightarrow{F_{1}}$ and $\overrightarrow{F_{3}}$ are on the same straight line.
Thus, Resultant force $=\overrightarrow{F_{3}} \overrightarrow{F_{1}}$ [ because $\overrightarrow{F_{3}}, \overrightarrow{F_{1}}$ ] $\overrightarrow{F_{A}}=6 \mathrm{~K}-2 \mathrm{~K}=4 \mathrm{~K}$
$\overrightarrow{F_{2}}$ and $\overrightarrow{F_{4}}$ are on the same straight line.
Resultant force $=F_{4}, F_{2}$ [because $F_{4} F_{2}$ ]
$\overrightarrow{F_{B}}=8 \mathrm{~K}-4 \mathrm{k}=4 \mathrm{~K}$
Total resultant force $\overrightarrow{F_{R}}=\sqrt{F_{A}^{2}+F_{B}^{2}+2 F_{A} F_{B} \cos \theta}$
Here $\cos \theta=0$ because $\theta=90^{\circ}$
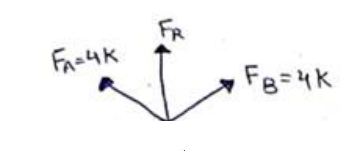
$\overrightarrow{F_{R}}=\sqrt{F_{A}^{2}+F_{B}^{2}}$
$=\sqrt{(4 K)^{2}+(4 K)^{2}}$
$=4 \sqrt{2} K$
$=4 \sqrt{2} \frac{G m^{2}}{a^{2}}$
