Three equal masses $\mathrm{m}$ are placed at the three corners of an equilateral triangle of side a. Find the force exerted by this system on another particle of mass $m$ placed at (a) the mid-point of a side, (b) at the center of the triangle.
Mass ' $m$ ' is at mid-point of side PR.
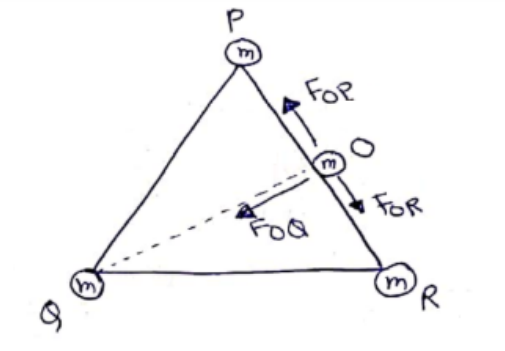
(a) OP $=\mathrm{OR}=\mathrm{a} / 2$
$\overline{F_{o p}}=\frac{G \times m \times m}{\left(a_{2}\right)^{2}} \quad \mathrm{~K}=\frac{\mathrm{Gm}^{2}}{a^{2}}$
$\overline{F_{O P}}=\frac{4 \mathrm{Gm}^{2}}{a^{2}}=4 \mathrm{~K}$
$\overline{F_{O R}}=\frac{4 \mathrm{G} m^{2}}{a^{2}}=4 \mathrm{~K}$
Altitude $\mathrm{OQ}=\frac{\sqrt{3}}{2} a$
$\overline{F_{O Q}}=\frac{\mathrm{Gm}^{2}}{\left(\frac{\sqrt{3}}{2} a\right)^{2}}=\frac{4 \mathrm{Gm}^{2}}{3 a^{2}}=\frac{4}{3} \mathrm{~K}$
Here, $F_{O P}$ and $F_{O R}$ are equal in magnitude and opposite in direction.
So, $F_{O P}+F_{O R}=0$
Total resultant force(F)
$=\overline{F_{O R}}+\overline{F_{O P}}+\overline{F_{O Q}}$
$=0+\overline{F_{O Q}}$
$=\overline{3} \mathrm{~K}$
$=\frac{4 \mathrm{G} \mathrm{m}^{2}}{3 a^{2}}$ (Along OQ)
(b) If particle at centroid, All forces 'F' are equal in magnitude.
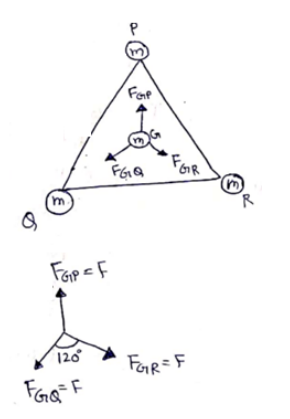
$F=F_{G P}=F_{G Q}=F_{G R}=\frac{3 G m^{2}}{a^{2}}$
Resultant of $F_{G Q}$ and $F_{G R}$
$\mathrm{F}_{\mathrm{R}=} \sqrt{F_{G Q}^{2}+F_{G R}^{2}-2 F_{G Q} \cdot F_{G R} \cdot \cos 120^{\circ}}$
$=\sqrt{F^{2}+F^{2}-2 F^{2} \cdot\left(\frac{1}{2}\right)}$
$\mathrm{F}_{\mathrm{R}}=\mathrm{F}$

The resultant is 0 , because they have equal magnitude and are in opposite direction.
